Rethinking Fractions

I have found that there is always more to learn when thinking about teaching fractions. At the recent COABE national conference, Melissa Braaten from the Adult Numeracy Center at TERC in Cambridge, MA, shared some compelling research about what community college developmental math students do not understand about mathematics and then offered some targeted classroom approaches based on the recommendations of that research. A key finding of that research was that many students were lacking an ability to reason about fractions and their values.

Here are some examples of types of questions that Melissa shared that students were missing:

1. Which is the largest:  4/7, 1/3, 3/8, or 4/9?
2. ¼ + 0.6 =
3. Which is larger, 4/5 or 5/8?

For each of these questions, diving right into finding the common denominator may not be the most efficient or useful strategy. These questions can be answered with reasoning, but only if the student has had experience reasoning with fractions and their values.

Working with benchmark fractions is one way to practice this reasoning. Benchmark fractions are familiar, friendly fractions like ½, ¼, or ¾. Benchmarks are an accessible topic that could work well in an English language classroom, in any class that explores charts and graphs, or in various levels of math classes.

A way to start guiding students to reason with benchmarks is to ask questions like:

• Will the answer be more than one or less than one?
• Is this amount more than half or less than half?
• Does this amount describe most of it or just a little bit?

In question number one above, students who have practiced reasoning about one half will be more likely to notice that only one of the four fractions is more than half, so that one must be the largest. How could an understanding of benchmarks help a student reason about the second and third questions?

Melissa shared a graphic that showed practical representations of benchmarks. Though you may be familiar with many of these representations, which ones are new to you? As you are designing lessons, where could you incorporate a few more of the representations? How are students reasoning about fractions in your classes?

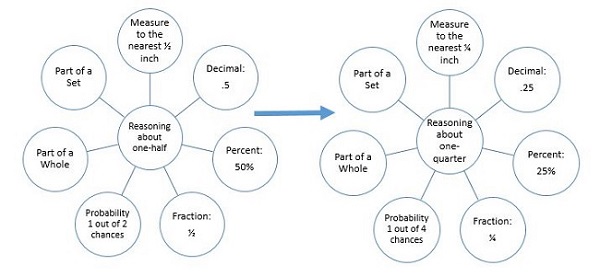
If you’d like to read more, here is the second part of the research that Melissa Shared: Part 2: The Interviews.